I once made the mistake of asking a mathematician why he devoted his whole life to maths. “Because it’s fun!” he replied wildly, his flabby cheeks beaming with childlike excitement.
“Ah, of course,” I thought to myself. “It is fun.”
But what can it do for society? It’s a question I’ve been asked by various people on scholarships committees and one that, over the course of this article and another to follow, I hope to answer.
I spend my time in an area of maths called number theory, which essentially looks at the shape of the counting numbers.
Other number theorists might encapsulate it differently, but it’s this perspective that keeps me on the edge of my seat. At the heart of this theory, we look at the distribution of the prime numbers.
Prime numbers
As one usually learns in their first few years of primary school, a prime number has a mathematical description (to do with factors) which, if stated here, might alienate more readers than it attracts. Let’s instead consider the following light-hearted definition:
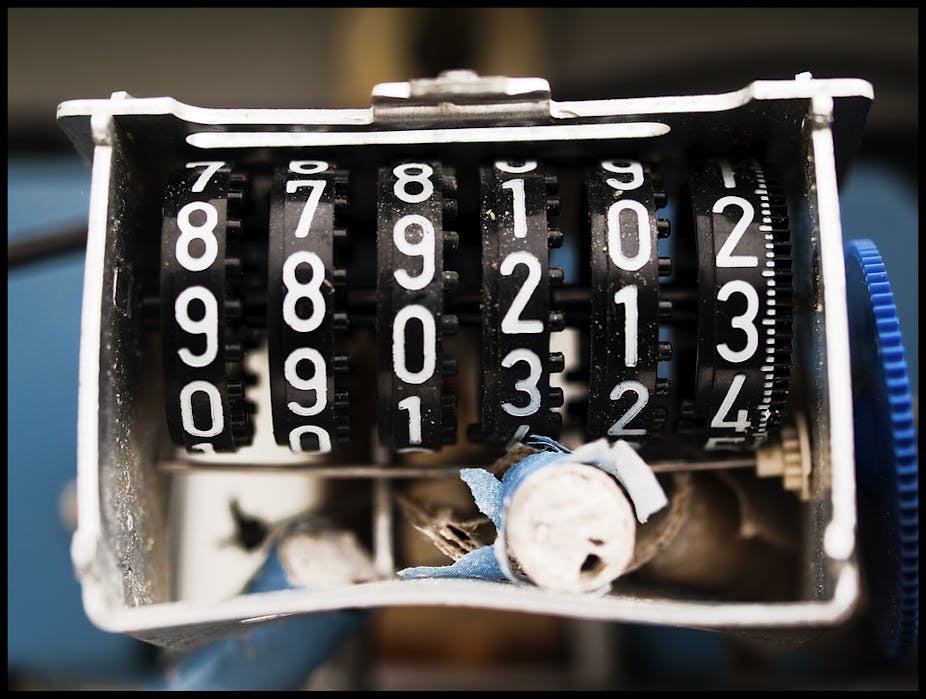
Let’s say you have a pile of biscuits. The number of biscuits you have is a prime number if there is no way to arrange those biscuits into a neat rectangle.
So if I gave you seven biscuits (think of it as a very early Christmas present) then you could arrange them like this:

We think of the above as a line, and not a rectangle. We could also arrange them like this:
But no matter how hard you try, you just won’t be able to arrange them into a rectangle! Therefore, seven is a prime number.
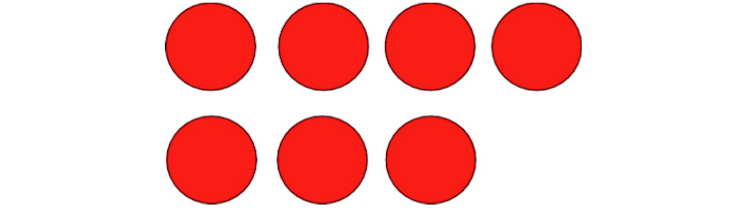
On the other hand, 12 is not a prime number, for 12 biscuits can be nicely arranged into a rectangle as follows:
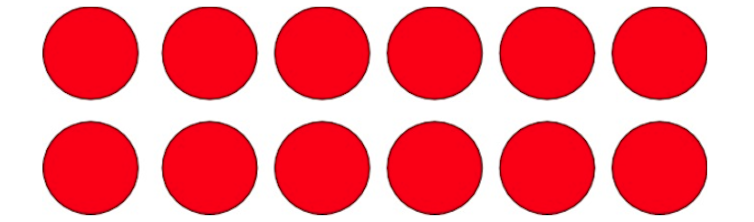
Or even like this:
If you wanted to, you could arrange 12 biscuits in a way that wasn’t a rectangle. The point is, if you can arrange them in a rectangle in at least one way, then the number is not a prime.
So there is something shape-y about the prime numbers. The really delightful thing is that prime numbers seem to just pop their head up in the counting numbers wherever they feel like it. Not only that, but they go on forever.
This fact – that there are infinitely many prime numbers – is an ancient theorem and was first proved to be true by the grand mathematician Euclid around 300 BC. The sequence of prime numbers starts as follows:
2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31 …
Primes are often called the atoms of arithmetic, and there is a good reason for this. Using only the primes, we can, through multiplication, build all of the composite (i.e. not prime) numbers.
Likewise, if we choose any counting number (except for 1, which is neither prime nor composite), then this number is either a prime or a bunch of primes multiplied together.
60 = 2 x 2 x 3 x 5
34 = 2 x 17
So why would the world’s mathematicians be interested in a subject that’s routinely covered in primary school?
The driving force here, as in most mathematics, is natural curiosity. As mentioned earlier, prime numbers, despite being very simple to describe, are tenacious in the way that they hide in the counting numbers.
That is, there is no identifiable pattern with which they appear.
In fact, questions concerning the distribution of prime numbers are among the hardest unsolved problems in all of mathematics.
A example from the top tier would be the twin prime conjecture, which asks if there are infinitely many primes which differ by only 2.
There are many instances of these twin prime pairs – such as 11 and 13, and 29 and 31 – but it is unknown whether there are infinitely many of these.
Number crunching
Some people believe the primes have been scattered randomly but others know better.
There are a stack of certified results that give order to the chaotic structure of the primes. One such result guarantees you can always find a prime between any number bigger than 1 and its double.
Another result ensures that given any fixed string of digits, there are infinitely many primes which contain this string. If you were to choose the string of digits “1234”, then there are infinitely many prime numbers which contain this string. Here are a few:
12343, 12347, 112349, 123401, 123407 …
The result does not tell you how to find these “string-containing primes”, only that infinitely many of them do exist. What an odd result! What if we were to choose, say, your mobile phone number for a string of digits?
Well, then there are infinitely many prime numbers which contain your phone number within their digits. Indeed, the prime numbers are rather intrusive!
It’s not at all surprising that many people have become mesmerised by the interplay between structure and randomness that presents itself within the sequence of prime numbers.
Some, they say, have even gone crazy trying to solve the difficult problems in the area. For most people, a more important problem seems to be the following: why should we care and is this useful maths?
The rift
Some people believe maths can be cleanly divided into two areas: useful maths and useless maths.
You could also be told that, as soon as a result has been used to model or measure some real-life scenario, it has been deemed useful.

In 1940, the renowned mathematician G. H. Hardy wrote A Mathematician’s Apology, an essay he hoped would deliver justification of his life’s work in mathematics.
Within this essay, Hardy labels number theory (his own area of mathematics) as useless. Indeed, at the time, number theory did not have any known uses, so I would have struggled even more with the scholarship committees of Hardy’s time.
But as all mathematicians know, the rift between useful and useless can change with time. In 1977, a trio of mathematicians found a permanent place for number theory in our modern world.
Ron Rivest, Adi Shamir and Leonard Adleman created the RSA algorithm (the three letters are taken from the initials of their surnames), as a way of transmitting secret information between two parties.
Why did they do this and what function does that algorithm play in the real world? That’s a question for the second, and final, part of this argument.
Read Adrian’s next article:
The RSA algorithm (or how to send private love letters)