I have spent almost 40 years trying to detect gravity waves.
When I started there were just a few of us working away in university labs. Today 1,000 physicists working with billion-dollar observatories are quietly confident the waves are within our grasp.
If we are right, the gravity wave search will have taken 100 years from the date of Einstein’s prediction.
In 100 years’ time the discovery of Einstein’s gravity waves will be one of the landmarks in the history of science. It will stand out like the discovery of electromagnetic waves in 1886, a quarter of a century after these waves were predicted by physicist James Clerk Maxwell.
The problem of talking about gravity waves is that you can’t explain them without explaining Einstein’s idea of gravity. Recently I began to ask why it is so difficult to explain gravity, why the concept is met with glazed eyes and baffled looks. Eventually I came up with a theory I call the Tragedy of the Euclidean Time Warp.
Discarding Euclidean ideas
My theory starts 2,300 years ago with the Greek mathematician Euclid’s book of geometry called Elements – the most influential book in the history of science.
Elements has been in print for more than 2,000 years and published in more than 1,000 editions. It was a basic school text for Galileo, Isaac Newton, Einstein and every educated person up to the baby-boomer generation. I still have the plain, slim edition that I used when I was in year eight.

The basic concepts from Elements are still taught in all primary schools and high schools throughout the world every day. We all know those concepts – parallel lines never meet, the sum of the angles of a triangle is 180º, the Theorem of Pythagoras and the perimeter formula for a circle (P = 2πr).
For centuries Euclidean geometry has moulded the way we think and today we all have an intuitive conception of space that is defined by Euclidean geometry.
The problem with Euclid’s book is that it cements a false idea about space. It is a shock to think that it is wrong but even so, gravity cannot be explained without discarding Euclidean geometry.
The link to General Relativity
The possibility of a flaw in Euclid’s book was first raised by the mathematician Carl Gauss in the 1820s. He published a theorem that said you could measure the shape of space by measuring angles and distances. He even tried to measure the shape of space on Earth by measuring the sum of the angles of a triangle between three mountain tops.
Some 90 years later, Einstein published his beautiful Theory of General Relativity, which gave us our current explanation for gravity. His theory is conceptually simple, but mathematically complex. Matter curves space and time, and gravity arises because of the way matter floats in this deformed space.
One of the key observations that confirmed the theory of curved space was made in Australia in 1922. The Wallal expedition obtained photos during a solar eclipse, from which the bending of the light as it passed by the sun were measured.
I think is rather shocking that today, 90 years later, we still teach geometry as if space were flat.
The reason our culture has not assimilated curved space is that we were all indoctrinated with Euclidean geometry during childhood. By the time we are adults it takes a painful re-think to adapt to a new way of thinking.

Trainee school teachers are rarely exposed to general relativity, so the teaching profession remains entirely free from Einstein’s beautiful theory. Generation after generation, this cycle continues. We are trapped in this Euclidean time warp. This is a tragedy not only because truth is important, but because students are disengaged by the stale teaching of obsolete 19th century physics.
A primary school experiment
Last year I tried to catch 11-year-olds before they were indoctrinated. Rosalie Primary School, in suburban Perth, agreed to host me, and I began six, weekly sessions with 30 primary school kids. Here is how we learnt that the force we call gravity arises because time is warped by matter.
First we talked about straight lines. How do we tell if lines are straight? Can you draw straight lines on balloons or the surface of the earth? What do surveyors do when they are building a straight fence? They always use sight lines, and when it comes down to it, straightness is always measured with light.
Then we considered drawing triangles on the earth. Suppose you start at the North Pole, travel south to the equator, turn left and travel 90º of longitude eastwards before taking another right turn to head back to the pole. We could all see that this triangle would have 90º + 90º + 90º – a total of 270º and definitely not what Euclid said.
Einstein said we should think about space and time together – what we call spacetime. But spacetime has four dimensions and we all agreed that our brains just don’t work properly for four dimensions.
So instead we agreed we could use just distance and time to keep it simple. We could then draw the spacetime diagram for the journey to school or for a water balloon falling from the Leaning Tower of Gingin, a full size steel replica of the Leaning Tower of Pisa, at an upcoming excursion.
Everyone could draw a spacetime diagram for their journey to school and point to the places where distance was not increasing but time was passing – waiting at the lights – and the steep bits when they were speeding down the freeway.
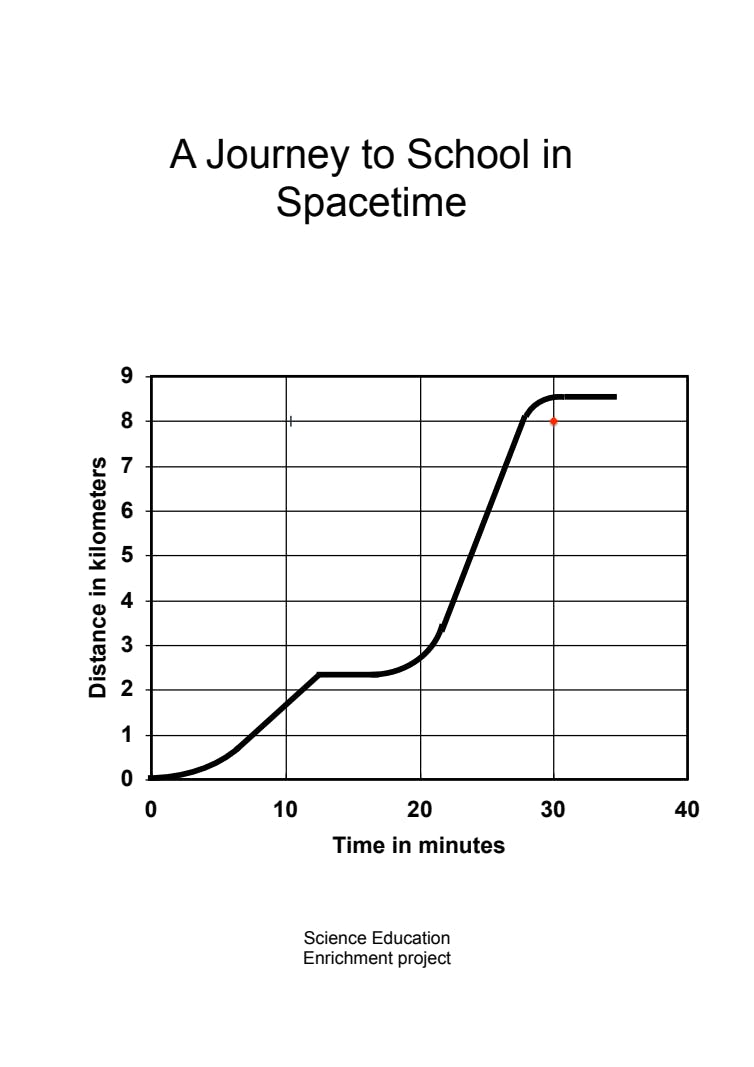
The next step in explaining Einstein’s theory of gravity is to think about the length of a trajectory in spacetime. Einstein said things in free-fall always have the shortest trajectory in spacetime. At first sight this is a weird idea. How can you measure a trajectory when one axis is distance and the other axis is time?
The journey-to-school diagram looks completely different if you change your units from seconds to minutes or meters to miles and the idea of the length of trajectory is pretty meaningless when both axes have different units.
The only sensible way to measure spacetime is to use a speed to enable us to measure time in space units. Using lightspeed as the conversion factor we can convert any time to the number of meters travelled by light in that time.
If you drop a water balloon from the Gingin Tower it takes almost three seconds to hit the ground. A spacetime diagram of its trajectory is a parabola that starts 45 meters above the ground, and hits the x-axis (time axis) three seconds later. But three seconds in time is 3 x 300,000km, or nine million meters.
The balloon travelled 900m meters in time. To full-scale the graph would stretch twice as far as the moon. The spacetime diagram is extremely elongated!
So now we can plot spacetime trajectories using meters for both distance and time, and I can now imagine using a tape measure to measure the length of any trajectory in meters.
Floating and falling in space
Now we come back to Einstein’s theory. It says that if you allow something to float freely in space, or fall from a tower, its trajectory in spacetime will always be the shortest.
Since a shortest line normally means a “straight line” Einstein is saying that free-floating trajectories are “straight lines” in spacetime (technically they are called geodesics).
Anything you do to prevent free-floating (like holding on to the water balloon on top of the tower rather than releasing it) will make the trajectory longer.

Now comes the Eureka moment. For the water balloon on the tower I want you to retort indignantly: “This is nonsense. It is obvious that the not-falling spacetime trajectory is always shorter than the falling trajectory!”
In one case the balloon fell down 45 meters, while in the other it did not even move in space, although it kept on going in time just as before. One trajectory was roughly diagonal on the spacetime graph but the other just moved parallel to the x-axis. This is like saying the hypotenuse of a right-angled triangle is shorter than its sides.
I reply: it is not nonsense! Einstein is correct because time depends on height above the earth. Time is warped. Time on the top of the tower is running 4 parts in one million billion times faster than it is on the ground.
Another way of saying this is that a clock on the top of the tower runs faster by four femtoseconds for every second. This is enough to stretch the time axis by just enough that not falling is actually longer than falling.
And there is yet another way of saying this. Gravity is the force you have to apply to objects to prevent them from freely floating in space. You do not feel gravity while you are falling because there is no force. You are just following the shortest path in spacetime.
What we call gravity is the result of the warping of time by the mass of the earth. Gravity is a force exerted by the earth to stop you from falling.
We all have a destination in spacetime that is called old age. Nature tries to make things get to this destination quickest.
An astronaut becomes an old astronaut quickest if he is floating around in the space station. The astronaut is higher above the surface of the earth than the top of the Gingin Tower. Time on the space station runs even faster than on top of the tower. Therefore the astronaut ages quicker there than on the earth’s surface or at the top of the tower. It takes forces to delay the progress of time.
We have a good planet that reliably provides continuous forces to us to prevent us free floating (i.e. falling) to the centre of the earth. So our planet is a time machine that delays our ageing (but just by a millisecond in a lifetime!).
The most astonishing thing about my program with Rosalie Primary School was that the kids were not astonished. My colleagues Grady Venville and Marina Pitts and I measured their learning and asked them if they thought they were too young to learn this stuff. By a large majority they thought they were not too young and they thought it was really interesting.
Physicists and astronomers deal with curved space every day, and even our GPS navigators have to correct for the warped spacetime around the earth. In May 2011, NASA’s Gravity Probe B spacecraft found that the perimeter of an orbit around the Earth failed to match its Euclidean value by 28 millimeters – not a large discrepancy but just the value predicted by Einstein.

Not too difficult to teach
In spite of modern science, the general belief among educators is that Einstein’s physics is too difficult to teach in school. As a result, science students enter university indoctrinated with 2,300-year-old Euclidean geometry and 300-year-old Newtonian physics.
Very few go on to discover the Einsteinian reality of curved space and warped time. The lucky few who get to study Einsteinian physics have difficulties because the fundamental concepts contradict all their past learning.
Most students who go on to become school teachers maintain the Newtonian mindset and so education remains in a Euclidean timewarp! The drastic decline in science at school and university could in part be due to our failure to challenge young people with modern ideas such as these.
If we start young enough, everyone can easily learn that the world is non-Euclidean, and then appreciate that the geometrical formulae we learn at school, such as Newton’s Law of Gravitation, are convenient approximations for everyday life.
See more Explainer articles on The Conversation.

