What do you get a mathematician for their birthday? Well, if it’s a big one – like a 100th – you could throw a conference in their honour. That’s what happened earlier this month, when mathematicians gathered in Hungary to mark 100 years since the birth of the late Paul Erdős.
During the meeting, the group discussed Erdős’s work as well as his legacy. Erdős was a remarkable man, both in terms of research style and mathematical ability. Let’s take a look at how he influenced the subject.

He was a problem solver
Broadly speaking, mathematics can be divided into two cultures. Some researchers focus mainly on solving problems, whereas others are more interested in developing and understanding theories. The problem solving side of the subject is usually the one people first encounter, and the one most often reported in the news. But many of the high profile areas of mathematics research concentrate on theory. Like in physics, attention and thus funding is given increasingly to grand, unifying ideas.
Erdős was more interested in the gaps between the theories. Awkward, unusual problems were his specialty, and he wrestled with puzzles from across the subject. He did not mind what tools he used: he was happy to attack problems with basic arithmetic or high school algebra. In doing so, Erdős showed that there are areas of mathematics that cannot be tamed by grand theories.
He published a lot of papers
Erdős’s hunt for interesting problems took him around the world. He was a mathematical nomad, living from two half-full suitcases without a chequebook or credit card. “Property is a nuisance,” as he put it.
He would turn up at colleagues’ houses and, fuelled by coffee and amphetamines, pick apart new riddles. His constant travels led to astonishing productivity: he published 1,525 papers during his life, a figure rivalled only by the great mathematician Leonhard Euler.
Although mathematics is often presented as a solitary subject, with breakthroughs coming from solo researchers, Erdős saw it as a social affair. The recent growth of polymath projects, with large groups tackling a particular problem, is a modern example of how successful Erdős’ collaborative philosophy can be.
He was well connected
By publishing and collaborating widely, Erdős ended up with over 500 co-authors. Fellow mathematicians have coined the idea of an Erdős number to measure how academically connected someone is to Erdős. If you published a paper with the man himself, you have an Erdős number of 1. If you wrote something with a person who published with Erdős, your number is 2, and so on. It’s the mathematical version of the “six degrees of Kevin Bacon”. (If you are a polymath, you can add your Bacon and Erdos numbers to get an “Erdős–Bacon number”: Richard Feynman’s is 6, as is Natalie Portman’s.)
As well as producing a hefty network of collaborations, Erdős also developed a neat way of generating artificial networks. The Erdős–Rényi model, design with fellow Hungarian Alfréd Rényi, was the first method for generating random graphs – networks in which there is an equal probability that any two nodes are connected.
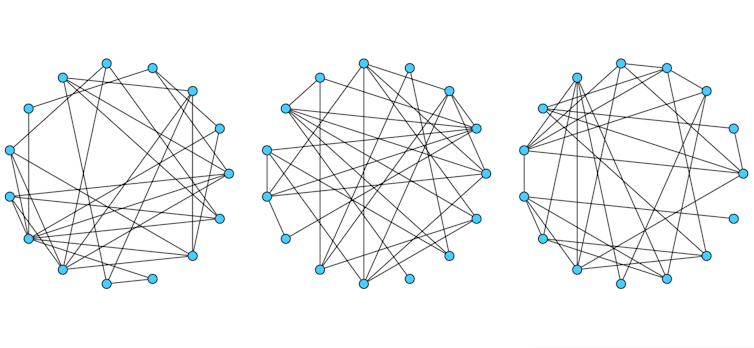
The mathematical simplicity of the Erdős–Rényi model means it is still used today, whether looking at the spread of epidemics or the stability of a financial system.
He liked prime numbers
Despite exploring other areas of mathematics, Erdős was always fascinated by prime numbers. In the late 1940s, his attention turned to the prime number theorem, which says that if you have a very large number – call it x – there are x/log(x) primes less than or equal to x (where log(x) is the natural logarithm). Earlier attempts to prove the Prime number theorem had been long and convoluted. But along with Norweigen mathematician Atle Selberg, Erdős found a far more elegant proof.
Erdős did not always manage to solve the problem he was working on. Although he chipped away at the twin prime conjecture – a solution for which was recently proposed by Yitang Zhang – Erdős could only prove a weaker, less definitive version of the theorem.

He offered rewards
To encourage people to tackle elusive problems, Erdős offered prizes for solutions. Problems that Erdős thought were fairly straightforward were worth $10 to $25, whereas complicated ones could have rewards running to thousands of dollars. It wasn’t the easiest way for mathematicians to make money: Erdős was notorious for reducing the prize if he did not like someone’s proof.
He didn’t win the biggest prize
Erdős may have offered prizes, but he did not always win them. After proving the prime number theorem with Erdős, Selberg rushed out a paper describing the solution. The work helped Selberg win the Fields Medal – the highest award in mathematics – but it was a prize that Erdős would never see.
Following the dispute with Selberg, Erdős packed his suitcases once again and continued his search for curious problems. Those travels, and the papers they produced, have left a long trail of ingenious methods and tantalising problems. Rényi once said “a mathematician is a machine for turning coffee into theorems”. And none did it nearly so well as Paul Erdős.
