The game show host adjusts his bow tie and flashes you an oh-so-wicked smile as he brings your attention to three closed doors.
“Behind one of these doors is the prize of your dreams!” he announces excitedly. “But oh - do choose wisely! There’s nothing to be won from choosing the other two doors.”
You think for some time until you decide there’s not really anything to think about in such a situation. It’s like flipping a coin, well, if coins had three sides. You take a deep breath and point to the door in the middle. “That one, please,” you say, almost confidently.
The host giggles and strolls over to the three doors. But instead of opening your door, he kicks open the door on the right, revealing nothing. “I’m going to be kind to you today,” he says through what could easily be the world’s widest smile. “As you can see, there is no prize behind the door on the right. I’m going to give you another choice. You can stick with the door you’ve chosen, or you can switch to the door on the left.”
You remember something that you were told once: always go with your gut instinct. “I’m going to stick with the door I’ve chosen,” you tell the host. “After all, it’s 50/50, right?”
Wrong. And here’s why.
The Monty Hall problem (and solution!)

The above scenario raises the following question: are you more likely to win if you switch from your original door of choice?
This question was put forward by American statistician Steve Selvin in a letter to the journal The American Statistician in 1975. It became known as the Monty Hall problem, named after the first host of the American television game show Let’s Make a Deal.
The problem notoriously rose to fame when it was presented in Marilyn vos Savant’s Ask Marilyn column in a 1990 issue of Parade magazine in the following form:
Suppose you’re on a game show, and you’re given the choice of three doors: behind one door is a car; behind the others, goats. You pick a door, say No. 1, and the host, who knows what’s behind the doors, opens another door, say No. 3, which has a goat. He then says to you, “Do you want to pick door No. 2?” Is it to your advantage to switch your choice?
Marilyn vos Savant, who rose to fame for once holding the Guinness world record in the “female with the highest IQ” category (before such a category was removed), said that in this scenario the contestant should always switch as by doing so they will be twice as likely to win than if they simply stuck with their original choice.
Her solution was met with fierce opposition; around 10,000 people wrote in (many of them academics) to dispute her assertion with the following logic:
If there are two closed doors left, one with a prize and one without, then you have an equal chance of winning seeing as you can choose which door to open.
But it’s now accepted that Marilyn’s solution is indeed correct - that is, you are twice as likely to win if you switch.
The explanation
Here we will see how the switching strategy will win the game two out of every three times. It will help if we can see what’s behind the doors, just for the sake of the explanation - and let’s assume you want to win a car rather than a goat.
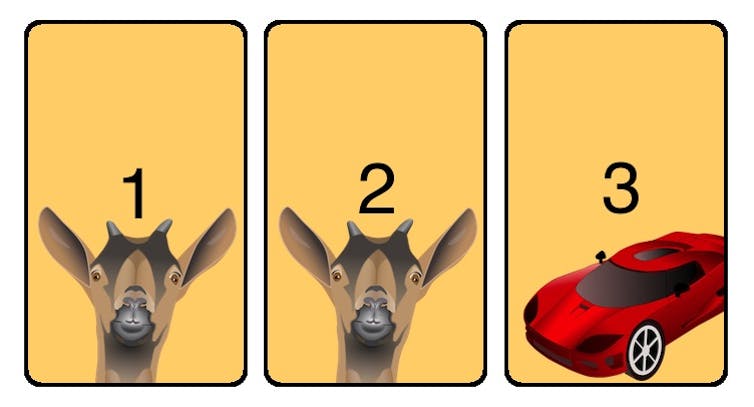
Case 1 If the contestant chooses door 1, then the game show host will reveal the goat behind door 2. In this case, switching will win the prize.
Case 2 If the contestant chooses door 2, then the game show host will reveal the goat behind door 1. In this case, switching always wins.
Case 3 If the contestant chooses door 3, then the game show host can show what’s behind either one of the other doors. Switching here will result in a goat rather than a car.
Therefore, we can see from this argument that switching always results in a win so long as the door we originally choose has a goat behind it. As this happens two-thirds of the time, it follows that we will acheive success two-thirds of the time by the switching strategy.
Still not convinced?
Sit down with a friend and simulate this, say with three upside-down cups and a marble under one of the cups as the prize. One of you plays the game show host and so knows what lies under each cup. The other plays the role of contestant and thus adopts the switching strategy.
Keep playing while recording how many times the contestant wins. You should find that after some time, the contestant will have about twice as many successes as losses.
Indeed, the prolific mathematician Paul Erdős remained unconvinced by all explanations. It was only when he watched the success-failure distribution given by a computer simulation that he came around.

